We'll explore this most basic analytical procedure of solving differential equations (DE): the separation of variables.
Separation of Variables
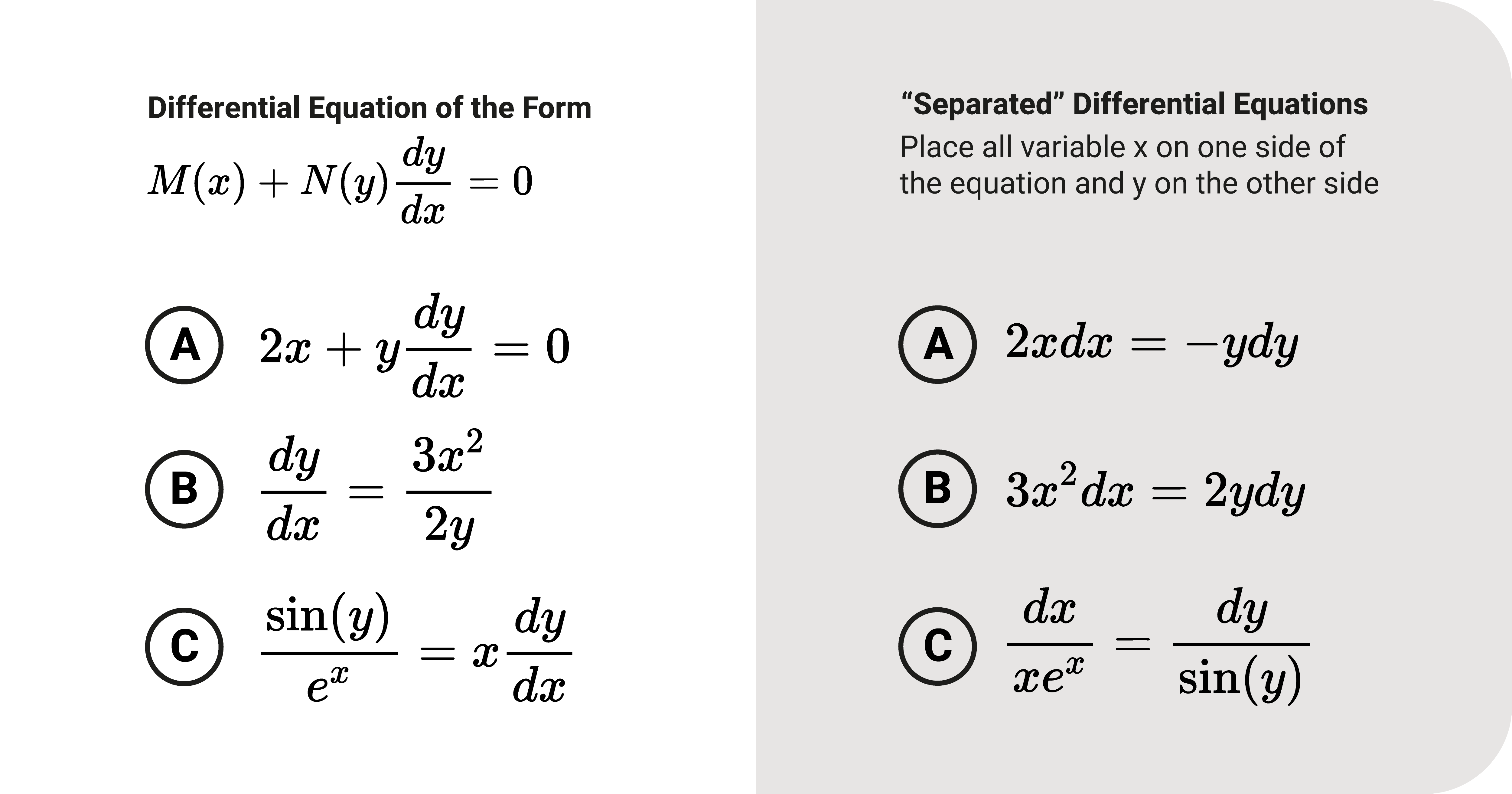
The separation of variables is the simplest method of finding solutions to a DE. It only works if the differential equation has the form: \(M(x) + N(y)y^\prime = 0\), where:
- \(M(x)\) is a function of \(x\)
- \(N(y)\) is a function of \(y\)
- \(\frac{dy}{dx}\) or \(y^\prime\) is the first derivative of \(N(y)\)
We can summarize the concept of this method using these steps:
- First, arrange all variables and differentials related to \(x\) on one side of the equation and those about \(y\) on the other. We can do this by transposing constants, variables, and differentials.
- After separating, integrate both sides of the equation to remove the differential; You'll end up with the solution to the DE.
Example
Consider the differential equation \(y^\prime = \frac{3x^2}{2y}\). Let's find the general solution to this DE:
Investigate DE
First, we investigate if the DE observes the form, \(M(x) + N(y)y^\prime = 0\).
Let's transpose the terms in our DE and try to match the form:
- \(y^\prime = \frac{3x^2}{2y}\)
- \(2y \cdot y^\prime = 3x^2\)
- \(3x^2 - 2y \cdot y^\prime = 0\)
In this rearranged form:
- \(M(x)\) is \(3x^2\)
- \(N(y)\) is \(2y\)
- \(y^\prime\) is \(y^\prime\)
It observes the general form; hence, our DE obeys the condition.
We can directly check if it obeys the form if we can separate the x-terms on one side and the y-terms on the other.
Apply Method
If the DE observes the form, we can use the separation of variables. Separate the variable \(x\) at one side of the equation and the variable \(y\):
- \(y^\prime = \frac{3x^2}{2y}\)
- \(\frac{dy}{dx} = \frac{3x^2}{2y}\)
- \(2ydy = 3x^2dx\)
Next, we integrate both sides of the equation to eliminate the differentials.
- \(\int 2ydy = \int 3x^2dx\)
- \(\frac{2 y^2}{2}=\frac{3 x^3}{3}+C_1\)
- \(y^2=x^3+C_1\)
The expression \(y^2=x^3+C_1\) is the general solution to the DE. To check your answer, substitute the solution and its derivatives to the original DE and determine if it satisfies the equation.
If there are conditions in the problem, we can expand our general solution to a particular one.
Constant of Integration
A thing of importance when it comes to integrating DE is the constant of integration \(C\). Ideally, you should include \(C\) every time you perform an indefinite integration. Since we performed two integrations, one on the left and another on the right side of the equation, we should have two constants on both sides; why do we have only one?
Supposedly we put two constants of integration. We let \(A\) and \(B\) be these constants on both sides, respectively. If we transpose both constants to one side, we'll combine their values \(A+B\). We represent this sum as \(C\). The constant of integration in a DE is already the combined value from both sides of the equation.
Summary
The separation of variables is the simplest method of finding solutions to a DE.
It only works if the differential equation has the form: \(M(x) + N(y)y^\prime = 0\), where: \(M(x)\) is a function of \(x\), \(N(y)\) is a function of \(y\), and \(\frac{dy}{dx}\), \(y^\prime\) is the first derivative of \(N(y)\)
First, arrange all variables and differentials related to \(x\) on one side of the equation and those about \(y\) on the other. We can do this by transposing constants, variables, and differentials.
After separating, integrate both sides of the equation to remove the differential; You'll end up with the solution to the DE.
A thing of importance when it comes to integrating DE is the constant of integration \(C\). It represents the combined constants from both sides of the equation after integrating.






