A body moving in a fluid will experience fluid resistance. In this post, we'll explore more about it.
Basics
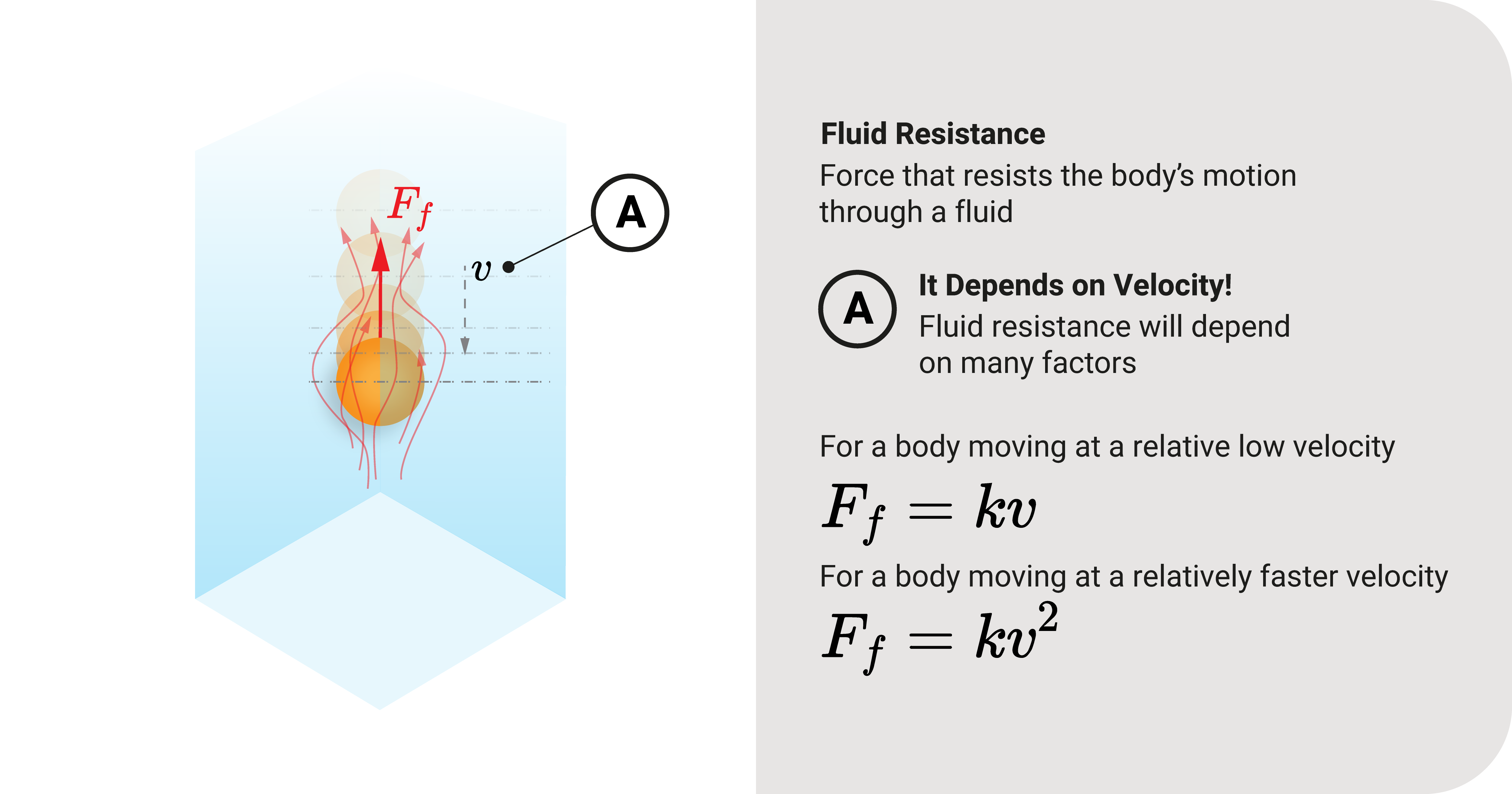
Whenever an object is moving in a fluid, there is a force that resists its motion. We call this fluid resistance \(F_f\). You can feel this when the wind slaps you when you are running around or if you stick your hands out of a fast-moving car.
Since this force opposes the motion, its direction will always be opposite to the body's movement. To be exact, it will be opposite to the velocity vector's direction \(v\). For example, if you fall from the sky, the fluid resistance is acting upwards - counteracting your fall.
It Depends on Velocity
Fluid resistance \(F_f\) will depend on the velocity of the moving body. There are two variations of \(F_f\):
- Case 1: Small objects moving through the fluid at a relatively low velocity \(v\)
- Case 2: Large objects moving through the fluid or an object moving through it at a relatively faster velocity \(v\)
Case 1
For a body moving with a relatively low velocity \(v\) through the fluid, such as a speck of dust falling in the air, \(F_f\) is directly proportional to it.
- \(F_f \propto v\)
- \(F_f = kv\); \(k\) is the constant of proportionality.
A good application of this resistance is Stokes' Law.
Case 2
For a large body moving through the fluid or an object moving at a relatively faster velocity \(v\) through it, such as a ball, rocket, or parachute in the sky, \(F_f\) is directly proportional to the square of the velocity \(v\)
- \(F_f \propto v^2\)
- \(F_f= kv^2\); \(k\) is the constant of proportionality.
A large object or an object moving faster will experience more resistance force than that of Case 1.
The constant \(k\) will depend on many factors, such as the body's size and shape and the fluid's density. We can find a variation of this expression through the Drag Equation.
Summary
Whenever an object is moving in a fluid, there is a force that resists its motion. We call this fluid resistance \(F_f\).
Since this force opposes the motion, its direction will always be opposite to the body's movement. To be exact, it will be opposite to the velocity vector's direction \(v\).
Fluid resistance \(F_f\) will depend on the velocity of the moving body.
There are two variations of \(F_f\): (1) Small objects moving through the fluid at a relatively low velocity \(v\), (2) Large objects moving through the fluid or an object moving through it at a relatively faster velocity \(v\)
For Case 1, \(F_f\) is directly proport\(F_f = kv\);
For Case 2, \(F_f\) is directly proportional to the square of the velocity \(v\): \(F_f = kv^2\)
The proportionality constant \(k\) will depend on many factors, such as the body's size and shape and the fluid's density.






